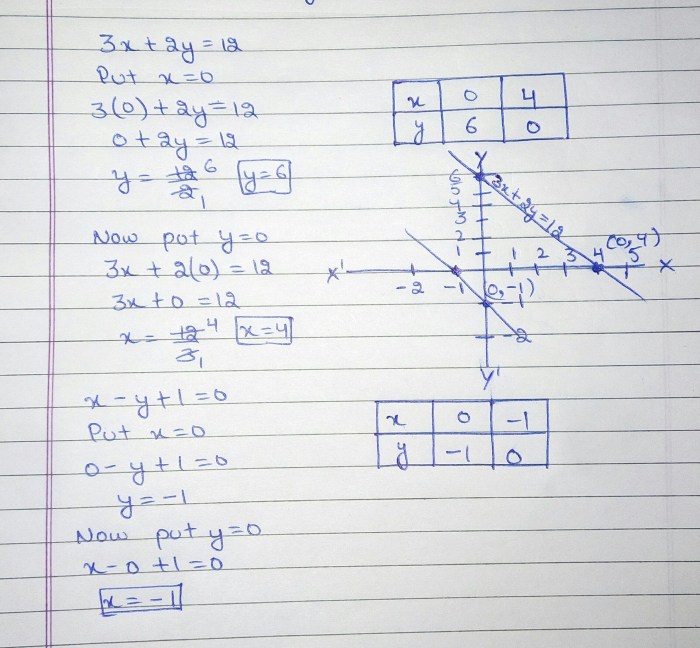
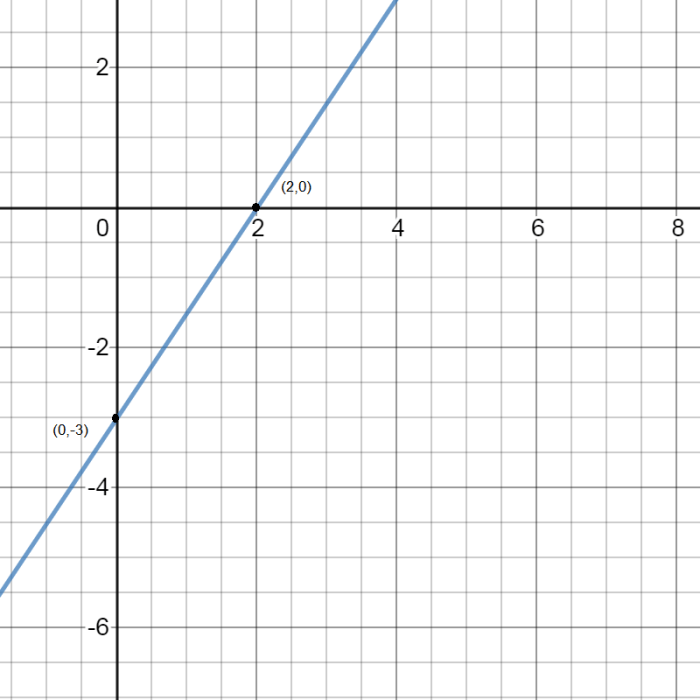Which is the graph of linear inequality 6x 2y – Delving into the realm of linear inequalities, we embark on an exploration of the graph of 6x + 2y. This mathematical concept finds its roots in the intersection of algebra and geometry, allowing us to represent inequalities graphically and analyze their solutions.
In this discourse, we will delve into the steps involved in graphing a linear inequality, decipher the different types of linear inequalities, and explore the concept of the solution region. Furthermore, we will examine real-world applications of linear inequalities, showcasing their practical significance in problem-solving and optimization.
Linear Inequality in Two Variables: Which Is The Graph Of Linear Inequality 6x 2y
A linear inequality in two variables is an inequality that can be written in the form Ax+ By≤ Cor Ax+ By≥ C, where A, B, and Care real numbers and xand yare variables.
The solution to a linear inequality is a point ( x, y) that makes the inequality true. The set of all solutions to a linear inequality is called the solution region.
Linear inequalities can be graphed on a coordinate plane. The boundary line of a linear inequality is the line that divides the plane into two half-planes. The solution region is the half-plane that contains the points that satisfy the inequality.
Graphing Linear Inequalities
To graph a linear inequality, follow these steps:
- Graph the boundary line. The boundary line is the line that represents the equation Ax+ By= C.
- Determine which side of the boundary line is the solution region. The solution region is the half-plane that contains the points that satisfy the inequality.
- Shade the solution region.
There are two types of linear inequalities: inequalities that are greater than or equal to (≥) and inequalities that are less than or equal to (≤).
- For an inequality that is greater than or equal to (≥), the solution region is the half-plane that is above the boundary line.
- For an inequality that is less than or equal to (≤), the solution region is the half-plane that is below the boundary line.
Specific Inequality: 6x + 2y, Which is the graph of linear inequality 6x 2y
Consider the inequality 6x + 2y ≤ 12.
The slope of the boundary line is -3, and the y-intercept is 6. To graph the boundary line, plot the point (0, 6) and use the slope to find another point on the line. The line should be dashed, since the inequality is ≤.
The solution region is the half-plane that is below the boundary line. Shade the solution region.
Solution Region
The solution region for the inequality 6x + 2y ≤ 12 is the half-plane that is below the boundary line.
A half-plane is a region of a plane that is bounded by a line. The boundary line divides the plane into two half-planes.
The solution region is the half-plane that contains the points that satisfy the inequality. In this case, the solution region is the half-plane that is below the boundary line because the inequality is ≤.
Applications of Linear Inequalities
Linear inequalities have many applications in real life.
- Linear inequalities can be used to solve problems in economics, business, and science.
- Linear inequalities can be used to model constraints and optimize solutions.
- Linear inequalities can be used to represent regions on a map.
Expert Answers
What is the slope of the boundary line for the inequality 6x + 2y?
-3
How do you determine the solution region for a linear inequality?
Shade the half-plane that contains the origin and satisfies the inequality.
What are some real-world applications of linear inequalities?
Modeling constraints in resource allocation, optimizing production schedules, and solving financial problems.